Electron. J. Differential Equations,
Vol. 2018 (2018), No. 121, pp. 1-36.
Nonlinear parabolic-elliptic system in Musielak-Orlicz-Sobolev spaces
Francisco Ortegon Gallego, Mohamed Rhoudaf, Hajar Sabiki
Abstract:
The existence of a capacity solution to the thermistor problem in the
context of inhomogeneous Musielak-Orlicz-Sobolev spaces is analyzed.
This is a coupled parabolic-elliptic system of nonlinear PDEs whose
unknowns are the temperature inside a semiconductor material,
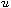 ,
and the electric potential,
,
and the electric potential,
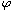 .
We study the general case where the nonlinear elliptic operator in the
parabolic equation is of the form
.
We study the general case where the nonlinear elliptic operator in the
parabolic equation is of the form
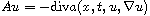 , A being a Leray-Lions operator
defined on
, A being a Leray-Lions operator
defined on
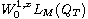 , where M is a generalized N-function.
, where M is a generalized N-function.
Submitted December 26, 2017. Published June 15, 2018.
Math Subject Classifications: 35J70, 35K61, 46E30, 35M13.
Key Words: Parabolic-elliptic system; Musielak-Orlicz-Sobolev spaces;
weak solutions; capacity solutions.
Show me the PDF file (437 KB),
TEX file for this article.
 |
Francisco Ortegón Gallego
Departamento de Matemáticas
Facultad de Ciencias, Universidad de Cádiz
Campus del Río San Pedro
11510 Puerto Real, Cádiz, Spain
email: francisco.ortegon@uca.es
|
|---|
 |
Mohamed Rhoudaf
Faculté des Sciences de Meknès
Université Moulay-Ismaïl - Meknès
Équipe: EDPs et Calcul Scientifique, Marocco
email: rhoudafmohamed@gmail.com
|
|---|
 |
Hajar Sabiki
Laboratoire d'Analyse
Géométrie et Applications
Faculté des Sciences
BP 133 Kénitra 14000, Marocco
email: sabikihajar@gmail.com
|
|---|
Return to the EJDE web page
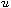 ,
and the electric potential,
,
and the electric potential,
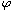 .
We study the general case where the nonlinear elliptic operator in the
parabolic equation is of the form
.
We study the general case where the nonlinear elliptic operator in the
parabolic equation is of the form
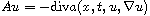 , A being a Leray-Lions operator
defined on
, A being a Leray-Lions operator
defined on
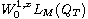 , where M is a generalized N-function.
, where M is a generalized N-function.


