Abhishek Sarkar
Abstract:
In this article we study the existence of at least two positive weak solutions
of an nonhomogeneous fourth-order Navier boundary-value problem involving
critical exponential growth on a bounded domain in
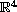 ,
with a parameter
,
with a parameter
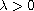 .
We establish upper and lower bounds for
.
We establish upper and lower bounds for
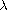 ,
which determine multiplicity and non-existence of solutions.
,
which determine multiplicity and non-existence of solutions.
Submitted September 12, 2016. Published January 24, 2017.
Math Subject Classifications: 35J30, 35J40, 35J60.
Key Words: Biharmonic; critical exponent; multiple solutions.
Show me the PDF file (297 KB), TEX file for this article.
 |
Abhishek Sarkar NTIS, University of West Bohemia Technicka 8, 306 14 Plzen Czech Republic email: sarkara@ntis.zcu.cz |
|---|
Return to the EJDE web page