Electron. J. Differential Equations,
Vol. 2017 (2017), No. 152, pp. 1-15.
Finite time blow-up of solutions for a nonlinear system
of fractional differential equations
Abdelaziz Mennouni, Abderrahmane Youkana
Abstract:
In this article we study the blow-up in finite time of solutions for
the Cauchy problem of fractional ordinary equations

for t>0, where the derivatives are Caputo fractional derivatives of order
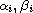 , and f and g are two continuously differentiable
functions with polynomial growth.
First, we prove the existence and uniqueness of local solutions
for the above system supplemented with initial conditions,
then we establish that they blow-up in finite time.
, and f and g are two continuously differentiable
functions with polynomial growth.
First, we prove the existence and uniqueness of local solutions
for the above system supplemented with initial conditions,
then we establish that they blow-up in finite time.
Submitted March 14, 2017. Published June 25, 2017.
Math Subject Classifications: 33E12, 34K37.
Key Words: Fractional differential equation; Caputo fractional derivative;
blow-up in finite time.
Show me the PDF file (254 KB),
TEX file for this article.
Abdelaziz Mennouni
Department of Mathematics
University of Batna 2
05078 Batna, Algeria
email: aziz.mennouni@yahoo.fr
|
Abderrahmane Youkana
Department of Mathematics
University of Batna 2
05078 Batna, Algeria
email: abder.youkana@yahoo.fr
|
Return to the EJDE web page

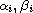 , and f and g are two continuously differentiable
functions with polynomial growth.
First, we prove the existence and uniqueness of local solutions
for the above system supplemented with initial conditions,
then we establish that they blow-up in finite time.
, and f and g are two continuously differentiable
functions with polynomial growth.
First, we prove the existence and uniqueness of local solutions
for the above system supplemented with initial conditions,
then we establish that they blow-up in finite time.