Electron. J. Diff. Equ.,
Vol. 2015 (2015), No. 168, pp. 1-16.
Existence and uniqueness of mild solutions for fractional semilinear
differential equations
Bambang Hendriya Guswanto, Takashi Suzuki
Abstract:
In this article, we study the existence and uniqueness of a local mild solution
for a class of semilinear differential equations involving the Caputo
fractional time derivative of order

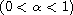 and, in the
linear part, a sectorial linear operator A. We put some conditions on a
nonlinear term f and an initial data
and, in the
linear part, a sectorial linear operator A. We put some conditions on a
nonlinear term f and an initial data
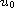 in terms of the fractional
power of A. By applying Banach's Fixed Point Theorem, we obtain a unique
local mild solution with smoothing effects, estimates, and a behavior at t
close to 0. An example as an application of our results is also given.
in terms of the fractional
power of A. By applying Banach's Fixed Point Theorem, we obtain a unique
local mild solution with smoothing effects, estimates, and a behavior at t
close to 0. An example as an application of our results is also given.
Submitted April 2, 2015. Published June 18, 2015.
Math Subject Classifications: 34A08, 34A12.
Key Words: Fractional semilinear differential equation; sectorial operator;
Caputo fractional derivative; fractional power; mild solution.
Show me the PDF file (263 KB),
TEX file, and other files for this article.
 |
Bambang Hendriya Guswanto
Department of Mathematics
Faculty of Mathematics and Natural Sciences
Jenderal Soedirman University (UNSOED)
Purwokerto, Indonesia
email: bambanghg_unsoed@yahoo.com; bambang.guswanto@unsoed.ac.id
|
|---|
 |
Takashi Suzuki
Division of Mathematical Science
Department of Systems Innovation
Graduate School of Engineering Science
Osaka University, Osaka, Japan
email: suzuki@sigmath.es.osaka-u.ac.jp
|
|---|
Return to the EJDE web page

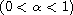 and, in the
linear part, a sectorial linear operator A. We put some conditions on a
nonlinear term f and an initial data
and, in the
linear part, a sectorial linear operator A. We put some conditions on a
nonlinear term f and an initial data
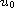 in terms of the fractional
power of A. By applying Banach's Fixed Point Theorem, we obtain a unique
local mild solution with smoothing effects, estimates, and a behavior at t
close to 0. An example as an application of our results is also given.
in terms of the fractional
power of A. By applying Banach's Fixed Point Theorem, we obtain a unique
local mild solution with smoothing effects, estimates, and a behavior at t
close to 0. An example as an application of our results is also given.

