Electron. J. Diff. Eqns., Vol. 2003(2003), No. 47, pp. 1-25.

Oscillation for equations with positive and negative coefficients and distributed delay II: Applications

Leonid Berezansky & Elena Braverman

Abstract:
We apply the results of our previous paper "Oscillation of equations with positive and negative coefficients and distributed delay I: General results" to the study of oscillation properties of equations with several delays and positive and negative coefficients
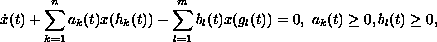
$$ \dot{x}(t) + \sum_{k=1}^n a_k(t) x(h_k(t)) - \sum_{l=1}^m b_l(t) x(g_l(t)) = 0, ~a_k(t) \geq 0, b_l(t) \geq 0, $$
integrodifferential equations with oscillating kernels and mixed equations combining two above equations. Comparison theorems, explicit non-oscillation and oscillation results are presented.

Submitted February 14, 2003. Published April 24, 2003.
Math Subject Classifications: 34K11, 34K15.
Key Words: Oscillation, non-oscillation, distributed delay, equations with several delays, integrodifferential equations.

Show me the PDF file (272K), TEX file, and other files for this article.

A related article has been published by the same authors in this journal: Oscillation for equations with positive and negative coefficients and with distributed delay I: General results, Vol. 2003(2003), No. 12, pp. 1-21.

Leonid Berezansky
Department of Mathematics
Ben-Gurion University of the Negev
Beer-Sheva 84105, Israel
e-mail: brznsky@cs.bgu.ac.il
Elena Braverman
Department of Mathematics and Statistics
University of Calgary
2500 University Drive N. W.,
Calgary, Alberta, Canada, T2N 1N4
Fax: (403)-282-5150, phone: (403)-220-3956
email: maelena@math.ucalgary.ca

Return to the EJDE web page