Eighth Mississippi State - UAB Conference on Differential Equations
and Computational Simulations.
Electron. J. Diff. Eqns., Conference 19 (2010), pp. 31-36.
Models of learning and the polar decomposition of
bounded linear operators
Fernanda Botelho, Annita Davis
Abstract:
We study systems of differential equations in
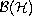 , the space of all bounded linear
operators on a separable complex Hilbert space
, the space of all bounded linear
operators on a separable complex Hilbert space
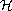 equipped with the operator norm. These systems are infinite
dimensional generalizations of mathematical models of learning.
We use the polar decomposition of operators to find an explicit
form for solutions. We also discuss the standard questions of
existence and uniqueness of local and global solutions, as well
as their long-term behavior.
equipped with the operator norm. These systems are infinite
dimensional generalizations of mathematical models of learning.
We use the polar decomposition of operators to find an explicit
form for solutions. We also discuss the standard questions of
existence and uniqueness of local and global solutions, as well
as their long-term behavior.
Published September 25, 2010.
Math Subject Classifications: 34G20, 47J25.
Key Words: Nonlinear systems; learning models;
polar decomposition of operators.
Show me the PDF file (173K),
TEX file, and other files for this article.
 |
Fernanda Botelho
The University of Memphis,
Mathematical Sciences Dept.
Dunn Hall 373, 3721 Norriswood St.
Memphis, TN 38152-3240, USA
email: mbotelho@memphis.edu
|
|---|
 |
Annita Davis
The University of Memphis,
Mathematical Sciences Dept.
Dunn Hall 373, 3721 Norriswood St.
Memphis, TN 38152-3240, USA
email: adavis2@memphis.edu
|
|---|
Return to the table of contents
for this conference.
Return to the EJDE web page
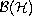 , the space of all bounded linear
operators on a separable complex Hilbert space
, the space of all bounded linear
operators on a separable complex Hilbert space
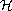 equipped with the operator norm. These systems are infinite
dimensional generalizations of mathematical models of learning.
We use the polar decomposition of operators to find an explicit
form for solutions. We also discuss the standard questions of
existence and uniqueness of local and global solutions, as well
as their long-term behavior.
equipped with the operator norm. These systems are infinite
dimensional generalizations of mathematical models of learning.
We use the polar decomposition of operators to find an explicit
form for solutions. We also discuss the standard questions of
existence and uniqueness of local and global solutions, as well
as their long-term behavior.

