We study the Neumann boundary value problem for stationary radial solutions of a quasilinear Cahn-Hilliard model in a ball
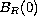 in
in
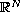 .
We establish new results on
the existence, uniqueness, and multiplicity (by "branching") of
such solutions. We show striking differences in pattern formation
produced by the Cahn-Hilliard model with the p-Laplacian and a
.
We establish new results on
the existence, uniqueness, and multiplicity (by "branching") of
such solutions. We show striking differences in pattern formation
produced by the Cahn-Hilliard model with the p-Laplacian and a
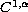 potential
(
potential
(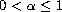 )
in place of the
regular (linear) Laplace operator and a
)
in place of the
regular (linear) Laplace operator and a
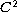 potential. The
corresponding energy functional exhibits one-dimensional
continua ("curves") of critical points as opposed to the
classical case with the Laplace operator. These facts offer a
different explanation of the "slow dynamics" on the attractor
for the dynamical system generated by the corresponding
time-dependent parabolic problem.
potential. The
corresponding energy functional exhibits one-dimensional
continua ("curves") of critical points as opposed to the
classical case with the Laplace operator. These facts offer a
different explanation of the "slow dynamics" on the attractor
for the dynamical system generated by the corresponding
time-dependent parabolic problem.
