Seventh Mississippi State - UAB Conference on Differential Equations and
Computational Simulations.
Electron. J. Diff. Eqns., Conference 17 (2009), pp. 95-105.
Infinitely many periodic solutions of nonlinear wave equations on $S^n$
Jintae Kim
Abstract:
The existence of time periodic solutions of nonlinear
wave equations

on
 -dimensional
spheres is considered. The corresponding
functional of the equation is studied by the convexity in
suitable subspaces, minimax arguments for almost symmetric
functional, comparison principles and Morse theory.
The existence of infinitely many time periodic solutions is
obtained where
-dimensional
spheres is considered. The corresponding
functional of the equation is studied by the convexity in
suitable subspaces, minimax arguments for almost symmetric
functional, comparison principles and Morse theory.
The existence of infinitely many time periodic solutions is
obtained where
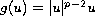 and the non-symmetric
perturbation
and the non-symmetric
perturbation
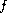 is not small.
is not small.
Published April 15, 2009.
Math Subject Classifications: 20H15, 20F18, 20E99, 53C55.
Key Words: Minimax theory; Morse index; critical points.
Show me the PDF file (245 KB),
TEX file, and other files for this article.
 |
Jintae Kim
Department of Mathematics, Tuskegee University
Tuskegee, AL 36088, USA
email: jtkim@tuskegee.edu
|
Return to the table of contents
for this conference.
Return to the EJDE web page

 -dimensional
spheres is considered. The corresponding
functional of the equation is studied by the convexity in
suitable subspaces, minimax arguments for almost symmetric
functional, comparison principles and Morse theory.
The existence of infinitely many time periodic solutions is
obtained where
-dimensional
spheres is considered. The corresponding
functional of the equation is studied by the convexity in
suitable subspaces, minimax arguments for almost symmetric
functional, comparison principles and Morse theory.
The existence of infinitely many time periodic solutions is
obtained where
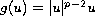 and the non-symmetric
perturbation
and the non-symmetric
perturbation
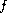 is not small.
is not small.
