2005-Oujda International Conference on Nonlinear Analysis,
Oujda, Morocco.
Electron. J. Diff. Eqns., Conference 14 (2006), pp. 73-81.
Leray Lions degenerated problem with general growth condition
Youssef Akdim, Abdelmoujib Benkirane, Mohamed Rhoudaf
Abstract:
In this paper, we study the existence of solutions for the
nonlinear degenerated elliptic problem
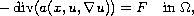
where
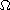 is a bounded domain of
is a bounded domain of
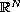 ,
,
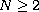 ,
,
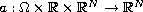 is
a Caratheodory function satisfying the coercivity condition,
but they verify the general growth condition and only the
large monotonicity. The second term
is
a Caratheodory function satisfying the coercivity condition,
but they verify the general growth condition and only the
large monotonicity. The second term
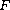 belongs to
belongs to
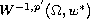 .
.
Published September 20, 2006.
Math Subject Classifications: 35J15, 35J70, 35J85.
Key Words: Weighted Sobolev spaces; truncations;
$L^1$-version of Minty's lemma; Hardy inequality.
Show me the
PDF file (204K),
TEX file, and other files for this article.
 |
Youssef Akdim
Département de Mathématiques et Informatique
Faculté des Sciences Dhar-Mahraz
B. P. 1796 Atlas Fès, Maroc
email: akdimyoussef@yahoo.fr |
|---|
 |
Abdelmoujib Benkirane
Département de Mathématiques et Informatique
Faculté des Sciences Dhar-Mahraz
B. P. 1796 Atlas Fès, Maroc
email: abenkirane@fsdmfes.ac.ma |
|---|
 |
Mohamed Rhoudaf
Département de Mathématiques et Informatique
Faculté des Sciences Dhar-Mahraz
B. P. 1796 Atlas Fès, Maroc
email: rhoudaf_mohamed@yahoo.fr |
|---|
Return to the table of contents for this conference.
Return to the EJDE web page
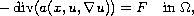
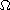 is a bounded domain of
is a bounded domain of
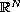 ,
,
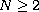 ,
,
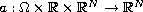 is
a Caratheodory function satisfying the coercivity condition,
but they verify the general growth condition and only the
large monotonicity. The second term
is
a Caratheodory function satisfying the coercivity condition,
but they verify the general growth condition and only the
large monotonicity. The second term
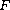 belongs to
belongs to
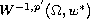 .
.


