The free undamped infinitesimal transverse vibrations of a thin straight beam are modelled by a forth-order differential equation. This paper investigates the families of fourth-order systems which have one spectrum in common, and correspond to four different sets of end-conditions. The analysis is based on the transformation of the beam operator into a fourth-order self-adjoint linear differential operator. This operator is factorized as a product
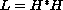 ,
where
,
where
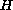 is a second-order differential operator of the form
is a second-order differential operator of the form
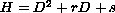 ,
and
,
and
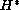 is its adjoint operator.
is its adjoint operator.
