2002-Fez conference on Partial Differental Equations,
Electron. J. Diff. Eqns., Conf. 09, 2002, pp. 25-39.
Strongly nonlinear degenerated elliptic unilateral problems
via convergence of truncations
Youssef Akdim, Elhoussine Azroul & Abdelmoujib Benkirane
Abstract:
We prove an existence theorem for a strongly nonlinear
degenerated elliptic inequalities involving nonlinear
operators of the form
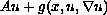 .
Here
.
Here
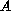 is a Leray-Lions operator,
is a Leray-Lions operator,
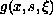 is a
lower order term satisfying some natural growth with respect
to
is a
lower order term satisfying some natural growth with respect
to
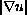 .
There is no growth restrictions with respect to
.
There is no growth restrictions with respect to
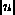 , only a sign condition. Under the assumption that the
second term belongs to
, only a sign condition. Under the assumption that the
second term belongs to
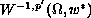 , we obtain
the main result via strong convergence of truncations.
, we obtain
the main result via strong convergence of truncations.
Published December 28, 2002.
Subject classfications: 35J15, 35J70, 35J85.
Key words: Weighted Sobolev spaces, Hardy inequality,
variational ineqality, strongly nonlinear
degenerated elliptic operators, truncations.
Show me the
PDF file (231K),
TEX file, and other files for this article.
 |
Youssef Akdim
Departement de Mathematiques et Informatique,
Faculte des Sciences Dhar-Mahraz,
B.P. 1796 Atlas, Fes, Maroc.
e-mail: y.akdim1@caramail.com |
|---|
 |
Elhoussine Azroul
Departement de Mathematiques et Informatique,
Faculte des Sciences Dhar-Mahraz,
B.P. 1796 Atlas, Fes, Maroc.
e-mail: elazroul@caramail.com |
|---|
 |
Abdelmoujib Benkirane
Departement de Mathematiques et Informatique,
Faculte des Sciences Dhar-Mahraz,
B.P. 1796 Atlas, Fes, Maroc.
e-mail: abdelmoujib@iam.net.ma |
|---|
Return to the table of contents
for this conference.
Return to the Electronic Journal of Differential Equations
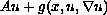 .
Here
.
Here
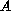 is a Leray-Lions operator,
is a Leray-Lions operator,
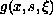 is a
lower order term satisfying some natural growth with respect
to
is a
lower order term satisfying some natural growth with respect
to
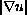 .
There is no growth restrictions with respect to
.
There is no growth restrictions with respect to
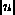 , only a sign condition. Under the assumption that the
second term belongs to
, only a sign condition. Under the assumption that the
second term belongs to
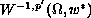 , we obtain
the main result via strong convergence of truncations.
, we obtain
the main result via strong convergence of truncations.


