USA-Chile Workshop on Nonlinear Analysis,
Electron. J. Diff. Eqns., Conf. 06, 2001, pp. 243-255.
On a fourth order superlinear elliptic problem
M. Ramos & P. Rodrigues
Abstract:
We prove the existence of a nonzero solution for the fourth order
elliptic equation
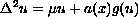
with boundary conditions
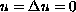 .
Here,
.
Here,
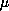 is a real parameter,
is a real parameter,
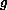 is superlinear
both at zero and infinity and
is superlinear
both at zero and infinity and
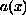 changes sign in
changes sign in
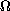 .
The proof uses a variational argument based on the argument
by Bahri-Lions [3].
.
The proof uses a variational argument based on the argument
by Bahri-Lions [3].
Published January 1, 2001.
Math Subject Classifications: 35J25, 35J20, 58E05.
Key Words: Superlinear elliptic problems, Morse index, biharmonic operator.
Show me the
PDF file (152K),
TEX file, and other files for this article.
Miguel Ramos
CMAF, Universidade de Lisboa
Av. Prof. Gama Pinto, 2
1649-003 Lisboa, Portugal
e-mail: mramos@lmc.fc.ul.pt
Paula Rodrigues
FCT, Universidade Nova de Lisboa
Quinta da Torre
2825 Monte da Caparica, Portugal
e-mail: paula.p.rodrigues@netc.pt
Return to the EJDE web page
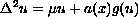
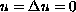 .
Here,
.
Here,
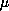 is a real parameter,
is a real parameter,
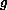 is superlinear
both at zero and infinity and
is superlinear
both at zero and infinity and
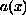 changes sign in
changes sign in
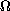 .
The proof uses a variational argument based on the argument
by Bahri-Lions [3].
.
The proof uses a variational argument based on the argument
by Bahri-Lions [3].