Electronic Journal of Differential Equations
15th annual Conference of Applied Mathematics, Univ. of Central Oklahoma,
Electron. J. Diff. Eqns., Conf. 02, 1999, pp. 47-60.
A one dimensional Hammerstein problem
Jun Hua & James L. Moseley
Abstract:
Nonlinear equations of the form
![$L[u]=\lambda g(u)$](gifs/aa.gif) where L is a linear
operator on a function space and g maps u to the composition
function
where L is a linear
operator on a function space and g maps u to the composition
function
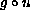 arise
in the theory of spontaneous combustion. If L is
invertible, such an equation can be written as a Hammerstein equation,
u=B[u] where
arise
in the theory of spontaneous combustion. If L is
invertible, such an equation can be written as a Hammerstein equation,
u=B[u] where
![$B[u]=\lambda L^{-1}[g(u)]$](gifs/ac.gif) . To investigate the importance of
the growth rate of g and the sign and magnitude of
. To investigate the importance of
the growth rate of g and the sign and magnitude of
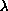 on the
number of solutions of such problems, in this paper we consider the
one-dimensional problem
on the
number of solutions of such problems, in this paper we consider the
one-dimensional problem
 where L(x)=ax.
where L(x)=ax.
Published November 24, 1999.
Subject Classification: 35P30.
Key words: Hammerstein problem, Nonlinear eigenvalue problem.
Show me the
PDF file (138K),
TEX file, and other files for this article.
 |
Jun Hua
West Virginia University
Morgantown, West Virginia 26506-6310 USA |
|---|
 |
James L. Moseley
West Virginia University
Morgantown, West Virginia 26506-6310 USA
e-mail: moseley@math.wvu.edu
Telephone: 304-293-2011 |
|---|
Return to the EJDE web page
![$L[u]=\lambda g(u)$](gifs/aa.gif) where L is a linear
operator on a function space and g maps u to the composition
function
where L is a linear
operator on a function space and g maps u to the composition
function
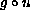 arise
in the theory of spontaneous combustion. If L is
invertible, such an equation can be written as a Hammerstein equation,
u=B[u] where
arise
in the theory of spontaneous combustion. If L is
invertible, such an equation can be written as a Hammerstein equation,
u=B[u] where
![$B[u]=\lambda L^{-1}[g(u)]$](gifs/ac.gif) . To investigate the importance of
the growth rate of g and the sign and magnitude of
. To investigate the importance of
the growth rate of g and the sign and magnitude of
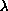 on the
number of solutions of such problems, in this paper we consider the
one-dimensional problem
on the
number of solutions of such problems, in this paper we consider the
one-dimensional problem
 where L(x)=ax.
where L(x)=ax. 
