Electron. J. Differential Equations,
Vol. 2018 (2018), No. 57, pp. 1-16.
Stability of traveling wavefronts for a three-component
Lotka-Volterra competition system on a lattice
Tao Su, Guo-Bao Zhang
Abstract:
This article concerns the stability of traveling wavefronts for a
three-component Lotka-Volterra competition system on a lattice.
By means of the weighted energy method and the comparison principle,
it is proved that the traveling wavefronts with large speed are
exponentially asymptotically stable, when the initial perturbation
around the traveling wavefronts decays exponentially as
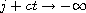 ,
where
,
where
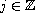 ,
,
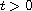 and
and
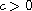 , but
the initial perturbation can be arbitrarily large on other locations.
, but
the initial perturbation can be arbitrarily large on other locations.
Submitted September 8, 2017. Published March 1, 2018.
Math Subject Classifications: 34A33, 34K20, 92D25.
Key Words: Three-component competition system; lattice dynamical system;
traveling wavefronts; stability.
Show me the PDF file (237 KB),
TEX file for this article.
 |
Tao Su
College of Mathematics and Statistics
Northwest Normal University
Lanzhou, Gansu 730070, China
email: 970913788@qq.com
|
|---|
 |
Guo-Bao Zhang
College of Mathematics and Statistics
Northwest Normal University
Lanzhou, Gansu 730070, China
email: zhanggb2011@nwnu.edu.cn
|
|---|
Return to the EJDE web page
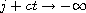 ,
where
,
where
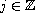 ,
,
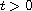 and
and
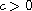 , but
the initial perturbation can be arbitrarily large on other locations.
, but
the initial perturbation can be arbitrarily large on other locations.

