Electron. J. Differential Equations,
Vol. 2018 (2018), No. 190, pp. 1-18.
Stability of weak solutions of a non-Newtonian polytropic filtration equation
Huashui Zhan, Zhaosheng Feng
Abstract:
We study a non-Newtonian polytropic filtration equation with a convection term.
We introduce new type of weak solutions and show the existence
of weak solutions. We show that when
![$\int_{\Omega} [a(x)]^{-1(p-1)}dx<\infty$](images/aa.png) ,
the stability of weak solutions is based on the usual initial-boundary
value conditions.
When
,
the stability of weak solutions is based on the usual initial-boundary
value conditions.
When
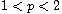 ,
under the given conditions on the diffusion coefficient and
the convection term, the stability of weak solutions can be proved without
any boundary value condition. In particular, the stability results are
presented based on the given optimal boundary value condition.
,
under the given conditions on the diffusion coefficient and
the convection term, the stability of weak solutions can be proved without
any boundary value condition. In particular, the stability results are
presented based on the given optimal boundary value condition.
Submitted March 21, 2018. Published November 26, 2018.
Math Subject Classifications: 35K20, 35K65, 35R35.
Key Words: Weak solution; convection term; stability; boundary value condition.
Show me the PDF file (292 KB),
TEX file for this article.
 |
Huashui Zhan
School of Applied Mathematics
Xiamen University of Technology
Xiamen, Fujian 361024, China
email: 2012111007@xmut.edu.cn
|
|---|
 |
Zhaosheng Feng
Department of Mathematics
University of Texas Rio Grande Valley
Edinburg, TX 78539, USA
email: zhaosheng.feng@utrgv.edu
|
|---|
Return to the EJDE web page
![$\int_{\Omega} [a(x)]^{-1(p-1)}dx<\infty$](images/aa.png) ,
the stability of weak solutions is based on the usual initial-boundary
value conditions.
When
,
the stability of weak solutions is based on the usual initial-boundary
value conditions.
When
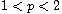 ,
under the given conditions on the diffusion coefficient and
the convection term, the stability of weak solutions can be proved without
any boundary value condition. In particular, the stability results are
presented based on the given optimal boundary value condition.
,
under the given conditions on the diffusion coefficient and
the convection term, the stability of weak solutions can be proved without
any boundary value condition. In particular, the stability results are
presented based on the given optimal boundary value condition.

