Electron. J. Differential Equations,
Vol. 2018 (2018), No. 142, pp. 1-21.
Existence of ground states for fractional Kirchhoff equations with
general potentials via Nehari-Pohozaev manifold
Jing Chen, Xianhua Tang, Sitong Chen
Abstract:
We consider the nonlinear fractional Kirchhoff equation
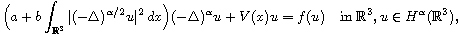
where a>0,
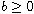 ,
,
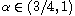 are three constants, V(x)
is differentiable and
are three constants, V(x)
is differentiable and
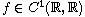 .
Our main results show the existence of ground state solutions of
Nehari-Pohozaev type, and the existence of the least energy solutions
to the above problem with general superlinear and subcritical nonlinearity.
These results are proved by applying variational methods and some techniques
from [27].
.
Our main results show the existence of ground state solutions of
Nehari-Pohozaev type, and the existence of the least energy solutions
to the above problem with general superlinear and subcritical nonlinearity.
These results are proved by applying variational methods and some techniques
from [27].
Submitted November 15, 2017. Published July 13, 2018.
Math Subject Classifications: 35J20, 35J65.
Key Words: Fractional Kirchhoff equation; Nehari-Pohozaev manifold;
ground state solutions.
Show me the PDF file (339 KB),
TEX file for this article.
 |
Jing Chen
School of Mathematics and Computing Sciences
Hunan University of Science and Technology
Xiangtan, Hunan 411201, China
email: cjhnust@aliyun.com
|
|---|
 |
Xianhua Tang
School of Mathematics and Statistics
Central South University
Changsha, 410083 Hunan, China
email: tangxh@mail.csu.edu.cn
|
|---|
 |
Sitong Chen
School of Mathematics and Statistics
Central South University
Changsha, 410083 Hunan, China
email: mathsitongchen@163.com
|
|---|
Return to the EJDE web page
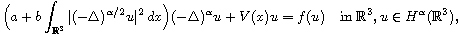
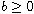 ,
,
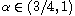 are three constants, V(x)
is differentiable and
are three constants, V(x)
is differentiable and
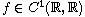 .
Our main results show the existence of ground state solutions of
Nehari-Pohozaev type, and the existence of the least energy solutions
to the above problem with general superlinear and subcritical nonlinearity.
These results are proved by applying variational methods and some techniques
from [27].
.
Our main results show the existence of ground state solutions of
Nehari-Pohozaev type, and the existence of the least energy solutions
to the above problem with general superlinear and subcritical nonlinearity.
These results are proved by applying variational methods and some techniques
from [27].


