Electron. J. Differential Equations,
Vol. 2018 (2018), No. 136, pp. 1-18.
Stability of an N-component Timoshenko beam with localized
Kelvin-Voigt and frictional dissipation
Tita K. Maryati, Jaime E. Munoz Rivera, Amelie Rambaud, Octavio Vera
Abstract:
We consider the transmission problem of a Timoshenko's beam composed by N
components, each of them being either purely elastic, or
a Kelvin-Voigt viscoelastic material, or an elastic material inserted with a
frictional damping mechanism.
Our main result is that the rate of decay depends on the
position of each component. More precisely, we prove that the
Timoshenko's model is exponentially stable if and only if all the
elastic components are connected with one component with frictional damping.
Otherwise, there is no exponential stability, but a polynomial decay of
the energy as
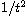 .
We introduce a new criterion to show the lack
of exponential stability, Theorem 1.2. We also consider the
semilinear problem.
.
We introduce a new criterion to show the lack
of exponential stability, Theorem 1.2. We also consider the
semilinear problem.
Submitted February 27, 2018. Published July 1, 2018.
Math Subject Classifications: 35B40, 74K10, 35M33, 35Q74.
Key Words: Timoshenko's model; beam equation; localized dissipation;
viscoelaticity; lack of exponential stability;
exponential and polynomial stability.
Show me the PDF file (303 KB),
TEX file for this article.
 |
Tita K. Maryati
Islamic State University (UIN)
Syarif Hidayatullah Jakarta, Indonesia
email: tita.khalis@uinjkt.ac.id
|
|---|
 |
Jaime E. Muñoz Rivera
Department of Mathematics
University of Bío-Bío
Concepción, Chile
email: jemunozrivera@gmail.com
|
|---|
 |
Amelie Rambaud
Department of Mathematics
University of Bío-Bío
Concepción, Chile
email: amelie.rambaud@yahoo.fr
|
|---|
 |
Octavio Vera
Department of Mathematics
University of Bío-Bío
Concepción, Chile
email: octaviovera49@gmail.com
|
|---|
Return to the EJDE web page
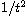 .
We introduce a new criterion to show the lack
of exponential stability, Theorem 1.2. We also consider the
semilinear problem.
.
We introduce a new criterion to show the lack
of exponential stability, Theorem 1.2. We also consider the
semilinear problem.



