We consider the following perturbed nonlinear elliptic problem with critical growth
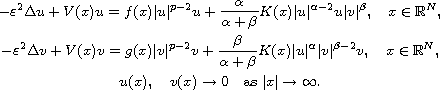
Using variational methods, we prove the existence of positive solutions.
Huixing Zhang, Ran Zhang
Abstract:
We consider the following perturbed nonlinear elliptic problem with critical
growth
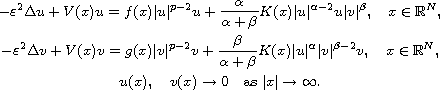
Using variational methods, we prove the existence of positive solutions.
Submitted April 20, 2016. Published February 21, 2017.
Math Subject Classifications: 35B33, 35J60, 35J65.
Key Words: Perturbed nonlinear Dirichlet problem; critical growth;
Palais-Smale condition; variational methods.
Show me the PDF file (240 KB), TEX file for this article.
 |
Huixing Zhang Department of Mathematics China University of Mining and Technology Xuzhou, Jiangsu 221116, China email: huixingzhangcumt@163.com |
|---|---|
 |
Ran Zhang Department of Mathematics China University of Mining and Technology Xuzhou, Jiangsu 221116, China email: ranzhang_zhangran@163.com |
Return to the EJDE web page