Electron. J. Differential Equations,
Vol. 2017 (2017), No. 53, pp. 1-11.
Stability of boundary-value problems for third-order partial
differential equations
Allaberen Ashyralyev, Kheireddine Belakroum, Assia Guezane-Lakoud
Abstract:
We consider a boundary-value problem for the third-order partial differential
equation
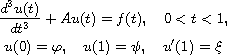
in a Hilbert space H with a self-adjoint positive definite operator A.
Using the operator approach, we establish stability estimates for
the solution of the boundary value problem. We study three types
of boundary value problems and obtain stability estimates for the solution
of these problems.
Submitted December 14, 2016. Published February 21, 2017.
Math Subject Classifications: 35G15, 47A62.
Key Words: Stability; boundary value problem; Hilbert space;
third order partial differential equation;
self-adjoint positive definite operator.
Show me the PDF file (224 KB),
TEX file for this article.
 |
Allaberen Ashyralyev
Department of Mathematics
Near East University, Nicosia, TRNC
Mersin 10, Turkey
email: allaberen.ashyralyev@neu.edu.tr
|
|---|
 |
Kheireddine Belakroum
Department of Mathematics
Frères Mentouri University
Constantine, Algeria
email: belakroumkheireddine@yahoo.com
|
|---|
 |
Assia Guezane-Lakoud
Laboratory of Advanced Materials
Mathematics Department, Faculty of Sciences
Badji Mokhtar Annaba University
P.O. Box 12, Annaba, 23000, Algeria
email: a_guezane@yahoo.fr
|
|---|
Return to the EJDE web page