In a recent book, the authors of this paper have studied the classical heat and Laplace equations with real time variable and complex spatial variable by the semigroup theory methods, under the hypothesis that the boundary function belongs to the space of analytic functions in the open unit disk and continuous in the closed unit disk, endowed with the uniform norm. The purpose of the present note is to show that the semigroup theory methods works for these evolution equations of complex spatial variables, under the hypothesis that the boundary function belongs to the much larger weighted Bergman space
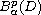 with
with
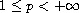 ,
endowed with a
,
endowed with a
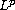 -norm.
Also, the case of several complex variables is considered. The
proofs require some new changes appealing to Jensen's inequality, Fubini's
theorem for integrals and the
-norm.
Also, the case of several complex variables is considered. The
proofs require some new changes appealing to Jensen's inequality, Fubini's
theorem for integrals and the
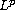 -integral modulus
of continuity. The
results obtained can be considered as complex analogues of those for the
classical heat and Laplace equations in
-integral modulus
of continuity. The
results obtained can be considered as complex analogues of those for the
classical heat and Laplace equations in
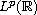 spaces.
spaces.

