Electron. J. Differential Equations,
Vol. 2017 (2017), No. 205, pp. 1-7.
Homoclinic solutions for a class of second-order
Hamiltonian systems with locally defined potentials
Xiang Lv
Abstract:
In this article, we establish sufficient conditions for the existence
of homoclinic solutions for a class of second-order Hamiltonian systems

where L(t) is a positive definite symmetric matrix for all
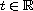 .
It is worth pointing out that the potential function W(t,u) is locally
defined and can be superquadratic or subquadratic with respect to u.
.
It is worth pointing out that the potential function W(t,u) is locally
defined and can be superquadratic or subquadratic with respect to u.
Submitted March 13, 2017. Published September 7, 2017.
Math Subject Classifications: 34C37, 70H05, 58E05.
Key Words: Homoclinic solutions; Hamiltonian systems; variational methods.
Show me the PDF file (206 KB),
TEX file for this article.
 |
Xiang Lv
Department of Mathematics
Shanghai Normal University
Shanghai 200234, China
email: lvxiang@shnu.edu.cn
|
|---|
Return to the EJDE web page

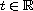 .
It is worth pointing out that the potential function W(t,u) is locally
defined and can be superquadratic or subquadratic with respect to u.
.
It is worth pointing out that the potential function W(t,u) is locally
defined and can be superquadratic or subquadratic with respect to u.
