Electron. J. Diff. Equ., Vol. 2016 (2016), No. 48, pp. 1-9.

Existence of solutions to differential inclusions with primal lower nice functions

Nora Fetouci, Mustapha Fateh Yarou

Abstract: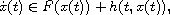
We prove the existence of absolutely continuous solutions to the differential inclusion
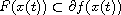$$ \dot{x}(t)\in F(x(t))+h(t,x(t)), $$
where F is an upper semi-continuous set-valued function with compact values such that $F(x(t))\subset \partial f(x(t))$ on [0,T], where f is a primal lower nice function, and h a single valued Caratheodory perturbation.

Submitted June 15, 2015. Published February 10, 2016.
Math Subject Classifications: 34A60, 49J52.
Key Words: Evolution problem; differential inclusion; primal lower nice functions; Caratheodory perturbation.

Show me the PDF file (207 KB), TEX file, and other files for this article.

  Nora Fetouci
LMPA Laboratory, Department of Mathematics
Jijel University, Algeria
email: norafetou2005@yahoo.fr
Mustapha Fateh Yarou
LMPA Laboratory, Department of Mathematics
Jijel University, Algeria
email: mfyarou@yahoo.com

Return to the EJDE web page