Electron. J. Differential Equations,
Vol. 2016 (2016), No. 250, pp. 1-20.
Self-similar solutions for a superdiffusive heat equation
with gradient nonlinearity
Marcelo Fernandes de Almeida, Arlucio Viana
Abstract:
This article studies the existence, stability, self-similarity and
symmetries of solutions for a superdiffusive heat equation with superlinear
and gradient nonlinear terms with initial data in new homogeneous Besov-Morrey
type spaces. Unlike in previous works on such time-fractional partial
differential equations of order
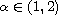 ,
we take non null initial
velocities into consideration, where new difficulties arise from.
We overcome them by developing an appropriate decomposition of the
two-parametric Mittag-Leffler function to obtain Mikhlin-type estimates
and obtain our existence theorem.
,
we take non null initial
velocities into consideration, where new difficulties arise from.
We overcome them by developing an appropriate decomposition of the
two-parametric Mittag-Leffler function to obtain Mikhlin-type estimates
and obtain our existence theorem.
Submitted July 8, 2016. Published Septembere 19, 2016.
Math Subject Classifications: 35A01, 35R11, 35R09, 35B06, 35C06, 35K05, 35L05.
Key Words: Fractional partial differential equations; self-similarity;
radial symmetry; Sobolev-Morrey spaces.
Show me the PDF file (342 KB),
TEX file for this article.
 |
Marcelo Fernandes de Almeida
Universidade Federal de Sergipe
DMA - Departamento de Matemática
Avenida Rosa Else, São Cristíovão
Sergipe, Brazil
email: nucaltiado@gmail.com
|
|---|
 |
Arlúcio Viana
Universidade Federal de Sergipe
DMAI - Departamento de Matemática
Avenida Vereador Olíimpio Grande
Itabaiana, Sergipe, Brazil
email: arlucioviana@ufs.br
|
|---|
Return to the EJDE web page
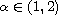 ,
we take non null initial
velocities into consideration, where new difficulties arise from.
We overcome them by developing an appropriate decomposition of the
two-parametric Mittag-Leffler function to obtain Mikhlin-type estimates
and obtain our existence theorem.
,
we take non null initial
velocities into consideration, where new difficulties arise from.
We overcome them by developing an appropriate decomposition of the
two-parametric Mittag-Leffler function to obtain Mikhlin-type estimates
and obtain our existence theorem.

