Electron. J. Differential Equations,
Vol. 2016 (2016), No. 243, pp. 1-10.
Lyapunov-type inequalities for odd order linear differential equations
Sougata Dhar, Qingkai Kong
Abstract:
In this article, we obtain Lyapunov-type inequalities for certain
odd order linear boundary-value problems. Our inequalities involve
integrals of both
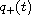 and
and
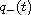 in addition to that of
in addition to that of
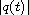 .
The Green's function for even order boundary-value problems plays a key
role in our proofs. Also, using the Fredholm alternative theorem,
we obtain a criterion for the existence and uniqueness of solutions to
the corresponding nonhomogeneous linear boundary-value problems.
.
The Green's function for even order boundary-value problems plays a key
role in our proofs. Also, using the Fredholm alternative theorem,
we obtain a criterion for the existence and uniqueness of solutions to
the corresponding nonhomogeneous linear boundary-value problems.
Submitted July 7, 2015. Published September 7, 2016.
Math Subject Classifications: 34A40, 34B05, 34C11, 26D20.
Key Words: Lyapunov-type inequalities; odd order; linear differential equations;
Green's function; boundary value problem.
Show me the PDF file (216 KB),
TEX file for this article.
 |
Sougata Dhar
Department of Mathematics
Northern Illinois University
DeKalb, IL 60115, USA
email: sdhar@niu.edu
|
|---|
 |
Qingkai Kong
Department of Mathematics
Northern Illinois University
DeKalb, IL 60115, USA
email: qkong@niu.edu
|
|---|
Return to the EJDE web page
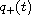 and
and
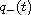 in addition to that of
in addition to that of
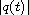 .
The Green's function for even order boundary-value problems plays a key
role in our proofs. Also, using the Fredholm alternative theorem,
we obtain a criterion for the existence and uniqueness of solutions to
the corresponding nonhomogeneous linear boundary-value problems.
.
The Green's function for even order boundary-value problems plays a key
role in our proofs. Also, using the Fredholm alternative theorem,
we obtain a criterion for the existence and uniqueness of solutions to
the corresponding nonhomogeneous linear boundary-value problems.

