Electron. J. Diff. Equ., Vol. 2016 (2016), No. 217, pp. 1-15.

Multiplicity of solutions for equations involving a nonlocal term and the biharmonic operator

Giovany M. Figueiredo, Rubia G. Nascimento

Abstract:
In this work we study the existence and multiplicity result of solutions to the equation
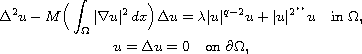
$$\displaylines{ \Delta^{2}u-M\Big(\int_{\Omega}|\nabla u|^{2} \,dx\Big)\Delta u = \lambda |u|^{q-2}u+ |u|^{2^{**}}u \quad\text{in }\Omega, \cr u=\Delta u=0 \quad\text{on }\partial\Omega, }$$
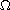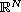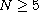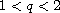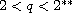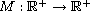where $\Omega$ is a bounded smooth domain of $\mathbb{R}^{N}$, $N\geq 5$, $1 < q<2$ or $2<q<2^{**}$, $M:\mathbb{R}^{+}\to\mathbb{R}^{+}$ is a continuous function. Since there is a competition between the function M and the critical exponent, we need to make a truncation on the function M. This truncation allows to define an auxiliary problem. 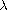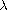We show that, for $\lambda$ large, exists one solution and for $\lambda$ small there are infinitely many solutions for the auxiliary problem. Here we use arguments due to Brezis-Niremberg [12] to show the existence result and genus theory due to Krasnolselskii [29] to show the multiplicity result. 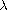Using the size of $\lambda$, we show that each solution of the auxiliary problem is a solution of the original problem.

Submitted April 20, 2016. Published August 16, 2016.
Math Subject Classifications: 34B15, 34B18. 35J35, 35G30
Key Words: Beam equation; Berger equation; critical exponent; variational methods.

Show me the PDF file (295 KB), TEX file for this article.

Giovany M. Figueiredo
Universidade Federal do Pará
Faculdade de Matemática
CEP: 66075-110 Belém - Pa, Brazil
email: giovany@ufpa.br
Rúbia G. Nascimento
Universidade Federal do Pará
Faculdade de Matemática
CEP: 66075-110 Belém - Pa, Brazil
email: rubia@ufpa.br

Return to the EJDE web page