Electron. J. Diff. Equ.,
Vol. 2016 (2016), No. 109, pp. 1-11.
Limit cycles bifurcated from a center in a three dimensional system
Bo Sang, Brigita Fercec, Qin-Long Wang
Abstract:
Based on the pseudo-division algorithm, we introduce a method for
computing focal values of a class of 3-dimensional autonomous systems.
Using the
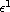 -order
focal values computation, we determine
the number of limit cycles bifurcating from each component of the center
variety (obtained by Mahdi et al). It is shown that at most four limit
cycles can be bifurcated from the center with identical quadratic
perturbations and that the bound is sharp.
-order
focal values computation, we determine
the number of limit cycles bifurcating from each component of the center
variety (obtained by Mahdi et al). It is shown that at most four limit
cycles can be bifurcated from the center with identical quadratic
perturbations and that the bound is sharp.
Submitted September 25, 2015. Published April 26, 2016.
Math Subject Classifications: 34C05, 34C07.
Key Words: Focal value; limit cycle; Hopf bifurcation; center.
Show me the PDF file (242 KB),
TEX file, and other files for this article.
 |
Bo Sang
School of Mathematical Sciences
Liaocheng University
email: sangbo_76@163.com
|
|---|
 |
Brigita Fercec
Faculty of Energy Technology
University of Maribor
Hocevarjev trg 1, 8270 Krsko, Slovenia
email: brigita.fercec@gmail.com
|
|---|
 |
Qin-Long Wang
School of Science
Hezhou University
Hezhou 542800, China
email: wqinlong@163.com
|
|---|
Return to the EJDE web page
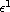 -order
focal values computation, we determine
the number of limit cycles bifurcating from each component of the center
variety (obtained by Mahdi et al). It is shown that at most four limit
cycles can be bifurcated from the center with identical quadratic
perturbations and that the bound is sharp.
-order
focal values computation, we determine
the number of limit cycles bifurcating from each component of the center
variety (obtained by Mahdi et al). It is shown that at most four limit
cycles can be bifurcated from the center with identical quadratic
perturbations and that the bound is sharp.


