In this article we consider the problem
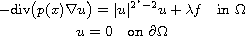
where
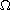 is a bounded domain in
is a bounded domain in
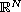 ,
We study the
relationship between the behavior of p near its minima on the
existence of solutions.
,
We study the
relationship between the behavior of p near its minima on the
existence of solutions.
Mohammed Bouchekif, Ali Rimouche
Abstract:
In this article we consider the problem
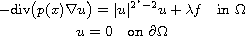
where
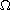 is a bounded domain in
is a bounded domain in
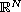 ,
We study the
relationship between the behavior of p near its minima on the
existence of solutions.
,
We study the
relationship between the behavior of p near its minima on the
existence of solutions.
Submitted June 30, 2015. Published January 6, 2016.
Math Subject Classifications: 35J20, 35J25, 35J60.
Key Words: Critical Sobolev exponent; Nehari manifold; variational principle.
Show me the PDF file (245 KB), TEX file for this article.
 |
Mohammed Bouchekif Laboratoire Systèmes Dynamiques et Applications Faculté des Sciences Université de Tlemcen BP 119 Tlemcen 13000, Algérie email: m_bouchekif@yahoo.fr |
|---|---|
 |
Ali Rimouche Laboratoire Systèmes Dynamiques et Applications Faculté des Sciences Université de Tlemcen BP 119 Tlemcen 13000, Algérie email: ali.rimouche@mail.univ-tlemcen.dz |
Return to the EJDE web page