In this article, we study the semilinear elliptic equation
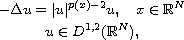
where
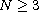 ,
,
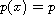 if
if
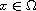 , and
, and
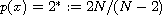 if
if
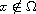 ,
where
,
where
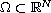 is a bounded
set with nonempty interior. By using the Nehari manifold, we obtain
a positive ground state solution.
is a bounded
set with nonempty interior. By using the Nehari manifold, we obtain
a positive ground state solution.
Jiu Liu, Jia-Feng Liao, Chun-Lei Tang
Abstract:
In this article, we study the semilinear elliptic equation
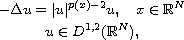
where
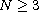 ,
,
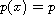 if
if
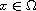 , and
, and
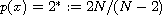 if
if
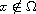 ,
where
,
where
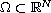 is a bounded
set with nonempty interior. By using the Nehari manifold, we obtain
a positive ground state solution.
is a bounded
set with nonempty interior. By using the Nehari manifold, we obtain
a positive ground state solution.
Submitted January 17, 2015. Published April 7, 2015.
Math Subject Classifications: 35J20, 35J61.
Key Words: Semilinear elliptic equation; zero mass; Nehari manifold;
ground state solution.
Show me the PDF file (236 KB), TEX file, and other files for this article.
 |
Jiu Liu School of Mathematics and Statistics Southwest University Chongqing 400715, China email: jiuliu2011@163.com |
|---|---|
 |
Jia-Feng Liao School of Mathematics and Statistics Southwest University Chongqing 400715, China email: liaojiafeng@163.com |
 |
Chun-Lei Tang School of Mathematics and Statistics Southwest University Chongqing 400715, China Phone +86 23 68253135, Fax +86 23 68253135 email: tangcl@swu.edu.cn |
Return to the EJDE web page