Uniqueness of self-similar very singular solutions with compact support are proved for the non-Newtonian polytropic filtration equation with gradient absorption
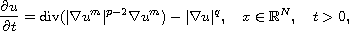
where
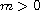 ,
,
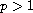 ,
,
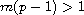 and
and
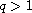 .
.
Hailong Ye, Jingxue Yin
Abstract:
Uniqueness of self-similar very singular solutions with compact support
are proved for the non-Newtonian polytropic filtration equation
with gradient absorption
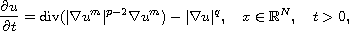
where
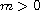 ,
,
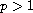 ,
,
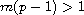 and
and
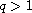 .
.
Submitted November 20, 2014. Published April 7, 2015.
Math Subject Classifications: 35K65, 35K92, 35K15.
Key Words: Polytropic filtration; gradient absorption; uniqueness; self-similar;
very singular.
Show me the PDF file (224 KB), TEX file, and other files for this article.
 |
Hailong Ye School of Mathematical Sciences South China Normal University Guangzhou, Guangdong 510631, China email: ye2006hailong@yeah.net |
|---|---|
 |
Jingxue Yin School of Mathematical Sciences South China Normal University Guangzhou, Guangdong 510631, China email: yjx@scnu.edu.cn |
Return to the EJDE web page