Electron. J. Diff. Equ.,
Vol. 2015 (2015), No. 70, pp. 1-10.
Oscillation of solutions to fourth-order trinomial delay
differential equations
Jozef Dzurina, Blanka Baculikova, Irena Jadlovska
Abstract:
The objective of this article is to study the oscillation properties of
the solutions to the fourth-order linear trinomial delay differential
equation
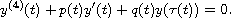
Applying suitable comparison principles, we present new criteria for
oscillation. In contrast with the existing results, we establish
oscillation of all solutions, and essentially simplify the examination
process for oscillation. An example is included to illustrate the
importance of results obtained.
Submitted February 5, 2015. Published March 24, 2015.
Math Subject Classifications: 34C10, 34K11.
Key Words: Fourth-order functional differential equation;
oscillation; delay argument; comparison theorem.
Show me the PDF file (187 KB),
TEX file, and other files for this article.
 |
Jozef Dzurina
Department of Mathematics
Faculty of Electrical Engineering and Informatics
Technical University of Kosice
Letna 9, 042 00 Kosice, Slovakia
email: jozef.dzurina@tuke.sk
|
|---|
 |
Blanka Baculikova
Department of Mathematics
Faculty of Electrical Engineering and Informatics
Technical University of Kosice
Letna 9, 042 00 Kosice, Slovakia
email: blanka.baculikova@tuke.sk
|
|---|
 |
Irena Jadlovska
Department of Mathematics
Faculty of Electrical Engineering and Informatics
Technical University of Kosice
Letna 9, 042 00 Kosice, Slovakia
email: irena.jadlovska@student.tuke.sk
|
|---|
Return to the EJDE web page