Electron. J. Diff. Equ.,
Vol. 2015 (2015), No. 295, pp. 1-7.
Existence and asymptotic behavior of positive solutions for
a second-order boundary-value problem
Ramzi S. Alsaedi
Abstract:
We study the boundary-value problem

where
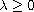 and f is nonnegative continuous and non-decreasing
with respect to the second variable. Under some assumptions on the
nonlinearity f, we prove the existence of a positive solution for
and f is nonnegative continuous and non-decreasing
with respect to the second variable. Under some assumptions on the
nonlinearity f, we prove the existence of a positive solution for
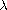 sufficiently small. Our approach is based on the Schauder
fixed point theorem.
sufficiently small. Our approach is based on the Schauder
fixed point theorem.
Submitted September 20, 2015. Published November 30, 2015.
Math Subject Classifications: 34B09, 34B15, 34B18, 34B27, 34B40.
Key Words: Boundary value problem; positive solution; fixed point;
Green function.
Show me the PDF file (188 KB),
TEX file, and other files for this article.
Ramzi S. Alsaedi
Department of Mathematics, Faculty of Sciences
King Abdulaziz University, P.O. Box 80203
Jeddah 21589, Saudi Arabia
email: ramzialsaedi@yahoo.co.uk
|
Return to the EJDE web page

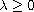 and f is nonnegative continuous and non-decreasing
with respect to the second variable. Under some assumptions on the
nonlinearity f, we prove the existence of a positive solution for
and f is nonnegative continuous and non-decreasing
with respect to the second variable. Under some assumptions on the
nonlinearity f, we prove the existence of a positive solution for
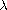 sufficiently small. Our approach is based on the Schauder
fixed point theorem.
sufficiently small. Our approach is based on the Schauder
fixed point theorem.