Electron. J. Diff. Equ.,
Vol. 2015 (2015), No. 270, pp. 1-19.
Remarks on the sharp constant for the Schrodinger Strichartz
estimate and applications
Alessandro Selvitella
Abstract:
In this article, we compute the sharp constant for the homogeneous
Schrodinger Strichartz inequality, and for the Fourier restriction
inequality on the paraboloid in any dimension under the condition
conjectured (and proved for dimensions 1 and 2) that the maximizers
are Gaussians. We observe also how this would imply a far from optimal,
but "cheap" and sufficient, criterion of the global wellposedness
in the
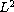 -critical case
-critical case
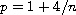 .
.
Submitted October 14, 2014. Published October 21, 2015.
Math Subject Classifications: 35Q41, 35A23.
Key Words: Strichartz estimate; optimal constant;
Schrodinger equation; restriction inequality.
Show me the PDF file (308 KB),
TEX file, and other files for this article.
 |
Alessandro Selvitella
Department of Mathematics & Statistics
McMaster University,
Hamilton Hall, Office 401HH
1280 Main Street West,
Hamilton, Ontario,
Canada L8S 4K1
email: aselvite@math.mcmaster.ca
|
|---|
Return to the EJDE web page
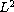 -critical case
-critical case
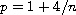 .
.
