This article concerns the Schrodinger equation
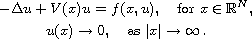
Assuming that V and f are periodic in x, and 0 is a boundary point of the spectrum
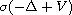 ,
two types of ground state solutions
are obtained with some super-quadratic conditions.
,
two types of ground state solutions
are obtained with some super-quadratic conditions.
Dongdong Qin, Xianhua Tang
Abstract:
This article concerns the Schrodinger equation
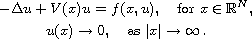
Assuming that V and f are periodic in x, and 0 is a boundary point
of the spectrum
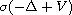 ,
two types of ground state solutions
are obtained with some super-quadratic conditions.
,
two types of ground state solutions
are obtained with some super-quadratic conditions.
Submitted March 2, 2015. Published July 20, 2015.
Math Subject Classifications: 35J10, 35J20.
Key Words: Schrodinger equation; spectrum point zero; superlinear;
ground states; Nehari-Pankov manifold.
Show me the PDF file (294 KB), TEX file, and other files for this article.
 |
Dongdong Qin School of Mathematics and Statistics Central South University Changsha, 410083 Hunan, China email: qindd132@163.com |
|---|---|
 |
Xianhua Tang School of Mathematics and Statistics Central South University Changsha, 410083 Hunan, China email: tangxh@mail.csu.edu.cn |
Return to the EJDE web page