Electron. J. Diff. Equ.,
Vol. 2015 (2015), No. 171, pp. 1-12.
Existence and asymptotic behavior of solutions to nonlinear radial
p-Laplacian equations
Syrine Masmoudi, Samia Zermani
Abstract:
This article concerns the existence, uniqueness and boundary behavior of
positive solutions to the nonlinear problem
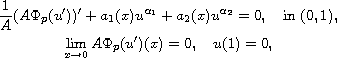
where
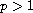 ,
,
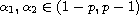 ,
,
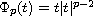 ,
,
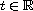 ,
,
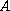 is a positive differentiable
function and
is a positive differentiable
function and
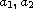 are two positive measurable functions in
are two positive measurable functions in
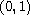 satisfying some assumptions related to Karamata regular
variation theory.
satisfying some assumptions related to Karamata regular
variation theory.
Submitted February 2, 2015. Published June 22, 2015.
Math Subject Classifications: 34B18, 35J66.
Key Words: p-Laplacian problem; positive solution; boundary behavior;
Schauder fixed point theorem
Show me the PDF file (254 KB),
TEX file, and other files for this article.
 |
Syrine Masmoudi
Département de Mathématiques,
Faculté des Sciences de Tunis
Campus Universitaire, 2092 Tunis, Tunisia
email: syrine.sassi@fst.rnu.tn
|
|---|
 |
Samia Zermani
Département de Mathématiques,
Faculté des Sciences de Tunis
Campus Universitaire, 2092 Tunis, Tunisia
email: zermani.samia@yahoo.fr
|
|---|
Return to the EJDE web page
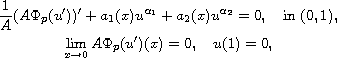
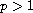 ,
,
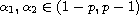 ,
,
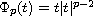 ,
,
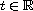 ,
,
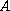 is a positive differentiable
function and
is a positive differentiable
function and
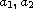 are two positive measurable functions in
are two positive measurable functions in
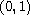 satisfying some assumptions related to Karamata regular
variation theory.
satisfying some assumptions related to Karamata regular
variation theory.

