We show that the system of difference equations
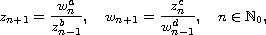
where
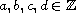 ,
and initial values
,
and initial values
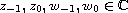 ,
is solvable in closed form, and present a method for finding its solutions.
,
is solvable in closed form, and present a method for finding its solutions.
Stevo Stevic, Mohammed A. Alghamdi,
Abdullah Alotaibi, Elsayed M. Elsayed
Abstract:
We show that the system of difference equations
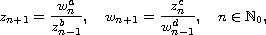
where
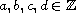 ,
and initial values
,
and initial values
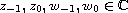 ,
is solvable in closed form, and present a method for finding its solutions.
,
is solvable in closed form, and present a method for finding its solutions.
Submitted February 7, 2015. Published June 18, 2015.
Math Subject Classifications: 39A10, 39A20.
Key Words: Solvable system of difference equations; second-order system;
product-type system; long-term behavior.
Show me the PDF file (321 KB), TEX file, and other files for this article.
 |
Stevo Stevic Mathematical Institute of the Serbian Academy of Sciences Knez Mihailova 36/III, 11000 Beograd, Serbia email: sstevic@ptt.rs |
|---|---|
| Mohammed A. Alghamdi Operator Theory and Applications Research Group Department of Mathematics, Faculty of Science King Abdulaziz University, P.O. Box 80203 Jeddah 21589, Saudi Arabia email: proff-malghamdi@hotmail.com | |
| Abdullah Alotaibi Operator Theory and Applications Research Group Department of Mathematics, Faculty of Science King Abdulaziz University, P.O. Box 80203 Jeddah 21589, Saudi Arabia email: aalotaibi@kau.edu.sa | |
| Elsayed M. Elsayed Department of Mathematics, Faculty of Science King Abdulaziz University, P.O. Box 80203 Jeddah 21589, Saudi Arabia email: emmelsayed@yahoo.com |
Return to the EJDE web page