This work concerns the unilateral problem for the Klein-Gordon operator
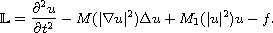
Using an appropriate penalization, we obtain a variational inequality for a perturbed equation, and then show the existence and uniqueness of solutions.
Carlos Raposo, Ducival Pereira, Geraldo Araujo, Antonio Baena
Abstract:
This work concerns the unilateral problem for the Klein-Gordon operator
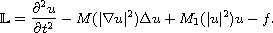
Using an appropriate penalization, we obtain a variational inequality for a
perturbed equation, and then show the existence and uniqueness of solutions.
Submitted July 29, 2014. Published May 20, 2015.
Math Subject Classifications: 35K60, 35F30.
Key Words: Unilateral problem; Kirchhoff-Carrier; Klein-Gordon equation;
weak solution; uniqueness of solutions.
Show me the PDF file (249 KB), TEX file, and other files for this article.
 |
Carlos Raposo Department of Mathematics, Federal University of São João Del-Rei São João Del-Rei - MG 36307-352, Brazil email: raposo@ufsj.edu.br |
|---|---|
 |
Ducival Pereira Department of Mathematics, State University of Pará Belém - PA 66113-200, Brazil email: ducival@oi.com.br |
 |
Geraldo Araujo Department of Mathematics, Federal University of Pará Belém - PA 66075-110, Brazil email: gera@ufpa.br |
 |
Antonio Baena Department of Mathematics, Federal University of Pará Belém - PA 66075-110, Brazil email: baena@ufpa.br |
Return to the EJDE web page