Electron. J. Diff. Equ., Vol. 2015 (2015), No. 127, pp. 1-11.

Super-quadratic conditions for periodic elliptic system on $\mathbb{R}^N$

Fangfang Liao, Xianhua Tang, Jian Zhang, Dongdong Qin

Abstract:
This article concerns the elliptic system
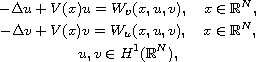
$$\displaylines{ -\Delta u+V(x)u=W_{v}(x, u, v), \quad x\in \mathbb{R}^{N},\cr -\Delta v+V(x)v=W_{u}(x, u, v), \quad x\in \mathbb{R}^{N},\cr u, v\in H^{1}(\mathbb{R}^{N}), }$$
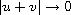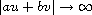where V and W are periodic in x, and W(x,z) is super-linear in z=(u,v). We use a new technique to show that the above system has a nontrivial solution under concise super-quadratic conditions. These conditions show that the existence of a nontrivial solution depends mainly on the behavior of W(x,u,v) as $|u+v| \to 0$ and $|au+bv| \to \infty$ for some positive constants a,b.

Submitted January 31, 2015. Published May 6, 2015.
Math Subject Classifications: 35J10, 35J20.
Key Words: Elliptic system; super-quadratic; nontrivial solution; strongly indefinite functionals.

Show me the PDF file (243 KB), TEX file, and other files for this article.

Fangfang Liao
School of Mathematics and Statistics
Central South University
Changsha, 41008, 3 Hunan, China
email: liaofangfang1981@126.com
Xianhua Tang
School of Mathematics and Statistics
Central South University
Changsha, 41008, 3 Hunan, China
email: tangxh@mail.csu.edu.cn
Jian Zhang
School of Mathematics and Statistics
Central South University
Changsha, 41008, 3 Hunan, China
email: zhangjian433130@163.com
Dongdong Qin
School of Mathematics and Statistics
Central South University
Changsha, 41008, 3 Hunan, China
email: qindd132@163.com

Return to the EJDE web page