Shapour Heidarkhani, Fariba Gharehgazlouei, Arezoo Solimaninia
Abstract:
In this article we study the existence of infinitely many radially
symmetric solutions for a class of perturbed elliptic equations
with discontinuous nonlinearities in
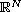 .
We determine open intervals of positive parameters for which the
problem admits infinitely many symmetric solutions. Our proofs are based on
variational methods.
.
We determine open intervals of positive parameters for which the
problem admits infinitely many symmetric solutions. Our proofs are based on
variational methods.
Submitted November 10, 2014. Published May 5, 2015.
Math Subject Classifications: 34A16, 35J20.
Key Words: Radially symmetric solutions; perturbed elliptic equation;
discontinuous nonlinearities; critical point theory; variational method.
Show me the PDF file (288 KB), TEX file, and other files for this article.
 |
Shapour Heidarkhani Department of Mathematics Faculty of Sciences, Razi University 67149 Kermanshah, Iran email: s.heidarkhani@razi.ac.ir |
|---|---|
 |
Fariba Gharehgazlouei Department of Mathematics Faculty of Sciences, Razi University 67149 Kermanshah, Iran email: f.gharehgazloo@yahoo.com |
 |
Arezoo Solimaninia Department of Mathematics Faculty of Sciences, Razi University 67149 Kermanshah, Iran email: a.solimaninia@yahoo.com |
Return to the EJDE web page