Electron. J. Diff. Equ.,
Vol. 2015 (2015), No. 114, pp. 1-16.
Structure of the solution set to differential inclusions with impulses
at variable times
Agata Grudzka, Sebastian Ruszkowski
Abstract:
A topological structure of the solution set to differential inclusions with
impulses at variable times is investigated. In order to do that an appropriate
Banach space is defined. It is shown that the solution set is an
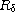 -set. Results are new also in the case of differential equations
with impulses at variable times.
-set. Results are new also in the case of differential equations
with impulses at variable times.
Submitted October 22, 2014 Published April 28, 2015.
Math Subject Classifications: 34A37, 34A60, 34K45.
Key Words: Solution set; impulsive differential inclusions;
variable times; R-delta-set; topological structure.
Show me the PDF file (262 KB),
TEX file, and other files for this article.
 |
Agata Grudzka
Faculty of Mathematics and Computer Science
Nicolaus Copernicus University
Chopina 12/18, 87-100 Torun, Poland
email: agata33@mat.uni.torun.pl
|
|---|
 |
Sebastian Ruszkowski
Faculty of Mathematics and Computer Science
Nicolaus Copernicus University
Chopina 12/18, 87-100 Torun, Poland
sebrus@mat.uni.torun.pl
|
|---|
Return to the EJDE web page
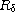 -set. Results are new also in the case of differential equations
with impulses at variable times.
-set. Results are new also in the case of differential equations
with impulses at variable times.

