This article concerns the entire solutions of a mono-stable age-structured population model in a 2D lattice strip. In a previous publication, we established the existence of entire solutions related to traveling wave solutions with speeds larger than the minimal wave speed
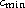 . However, the existence of entire solutions related to the minimal
wave fronts remains open open question.
In this article, we first establish a new comparison theorem.
Then, applying the theorem we obtain the existence of entire solutions by
mixing any finite number of traveling wave fronts with speeds
. However, the existence of entire solutions related to the minimal
wave fronts remains open open question.
In this article, we first establish a new comparison theorem.
Then, applying the theorem we obtain the existence of entire solutions by
mixing any finite number of traveling wave fronts with speeds
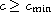 ,
and a solution without the j variable. In particular, we show the
relationship between the entire solution and the traveling wave fronts that
they originate.
,
and a solution without the j variable. In particular, we show the
relationship between the entire solution and the traveling wave fronts that
they originate.

