Electron. J. Diff. Equ.,
Vol. 2014 (2014), No. 226, pp. 1-9.
Nonuniqueness and fractional index convolution complementarity problems
David E. Stewart
Abstract:
Uniqueness of solutions of fractional index convolution complementarity
problems (CCPs) has been shown for index
 with
with
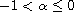 under mild assumptions, but not for
under mild assumptions, but not for
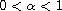 .
Here a family of
counterexamples is given showing that uniqueness generally fails for
.
Here a family of
counterexamples is given showing that uniqueness generally fails for
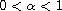 .
These results show that uniqueness is expected to fail
for convolution complementarity problems of the type that arise in
connection with solutions of impact problems for Kelvin-Voigt viscoelastic
rods.
.
These results show that uniqueness is expected to fail
for convolution complementarity problems of the type that arise in
connection with solutions of impact problems for Kelvin-Voigt viscoelastic
rods.
Submitted June 4, 2014. Published October 22, 2014.
Math Subject Classifications: 90C33, 74M20, 34A08.
Key Words: Convolution complementarity problem; mechanical impact;
viscoelasticity; uniqueness.
Show me the PDF file (213 KB),
TEX file, and other files for this article.
 |
David E. Stewart
Department of Mathematics,
University of Iowa
Iowa City, IA 52242, USA
email: david-e-stewart@uiowa.edu
|
|---|
Return to the EJDE web page
 with
with
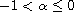 under mild assumptions, but not for
under mild assumptions, but not for
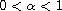 .
Here a family of
counterexamples is given showing that uniqueness generally fails for
.
Here a family of
counterexamples is given showing that uniqueness generally fails for
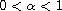 .
These results show that uniqueness is expected to fail
for convolution complementarity problems of the type that arise in
connection with solutions of impact problems for Kelvin-Voigt viscoelastic
rods.
.
These results show that uniqueness is expected to fail
for convolution complementarity problems of the type that arise in
connection with solutions of impact problems for Kelvin-Voigt viscoelastic
rods.
