Electron. J. Diff. Equ.,
Vol. 2014 (2014), No. 204, pp. 1-12.
Growth of solutions of linear differential equations with
analytic coefficients of [p,q]-order in the unit disc
Hui Hu, Xiu-Min Zheng
Abstract:
In this article, we study the growth of solutions of homogeneous
linear differential equation in which the coefficients are analytic
functions of [p,q]-order in the unit disc.
We obtain results about the (lower) [p,q]-order of the
solutions, and the (lower) [p,q]-convergence exponent for the
sequence of distinct zeros of
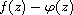 .
.
Submitted October 23, 2013. Published September 30, 2014.
Math Subject Classifications: 30D35, 34M10.
Key Words: Unit disc; analytic function; (lower) [p,q]-order;
(lower) [p,q]-type; (lower) [p,q]-convergence exponent.
Show me the PDF file (248 KB),
TEX file, and other files for this article.
 |
Hui Hu
Institute of Mathematics and Information Science
Jiangxi Normal University, 330022, China
email: h_h87_6@hotmail.com
|
|---|
 |
Xiu-Min Zheng
Institute of Mathematics and Information Science
Jiangxi Normal University, 330022, China
email: zhengxiumin2008@sina.com
|
|---|
Return to the EJDE web page
 .
.

