Electron. J. Diff. Equ.,
Vol. 2014 (2014), No. 137, pp. 1-14.
Minimization of energy integrals associated with the p-Laplacian
in R^N for rearrangements
Shanming Ji, Jingxue Yin, Rui Huang
Abstract:
In this article, we establish the existence of minimizers for energy
integrals associated with the p-Laplacian in
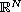 with the admissible set being a rearrangement class of a given function.
Some representation formulae of the minimizers are also stated.
with the admissible set being a rearrangement class of a given function.
Some representation formulae of the minimizers are also stated.
Submitted April 3, 2014. Published June 11, 2014.
Math Subject Classifications: 34K37, 46E35, 47H10.
Key Words: Sobolev space; periodic solution; locally Lipschitz potential;
AR-condition; nonsmooth C-condition;
the least action principle; mountain pass lemma.
Show me the PDF file (280 KB),
TEX file, and other files for this article.
 |
Shanming Ji
School of Mathematical Sciences
South China Normal University
Guangzhou 510631, China
email: sam@m.scnu.edu.cn
|
|---|
 |
Jingxue Yin
School of Mathematical Sciences
South China Normal University
Guangzhou 510631, China
email: yjx@scnu.edu.cn
|
|---|
 |
Rui Huang
School of Mathematical Sciences
South China Normal University
Guangzhou 510631, China
email: huang@scnu.edu.cn
|
|---|
Return to the EJDE web page
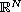 with the admissible set being a rearrangement class of a given function.
Some representation formulae of the minimizers are also stated.
with the admissible set being a rearrangement class of a given function.
Some representation formulae of the minimizers are also stated.


