Electron. J. Diff. Equ.,
Vol. 2013 (2013), No. 187, pp. 1-14.
The role of Riemann generalized derivative in the
study of qualitative properties of functions
Sorin Radulescu, Petrus Alexandrescu, Diana-Olimpia Alexandrescu
Abstract:
Marshal Ash [3] introduced the concept of
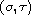 differentiable functions and studied the Riemann generalized derivatives
In this article we study the convexity and monotonicity of
differentiable functions and studied the Riemann generalized derivatives
In this article we study the convexity and monotonicity of
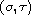 differentiable functions, using results by Hincin, Humke and Laczkovich,
and using the Riemann generalized derivative.
We give conditions such that the classic properties of differentiable
functions hold also for
differentiable functions, using results by Hincin, Humke and Laczkovich,
and using the Riemann generalized derivative.
We give conditions such that the classic properties of differentiable
functions hold also for
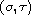 differentiable functions.
differentiable functions.
Submitted April 23, 2013. Published August 23, 2013.
Math Subject Classifications: 26A24, 28A15
Key Words: Riemann generalized derivative;
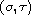 differentiable functions;
monotonicity; convexity; symmetric derivative; Schwarz derivative;
Darboux property.
differentiable functions;
monotonicity; convexity; symmetric derivative; Schwarz derivative;
Darboux property.
Show me the PDF file (226 KB),
TEX file, and other files for this article.
 |
Sorin Radulescu
Institute of Mathematical Statistics and Applied Mathematics
Calea 13 Septembrie, no. 13, Bucharest 5, RO-050711, Romania
email: xsradulescu@gmail.com
|
|---|
 |
Petrus Alexandrescu
Institute of sociology, Casa Academiei Romane
Calea 13 Septembrie, no. 13, Bucharest 5, RO-050711, Romania
email: alexandrescu_petrus@yahoo.com
|
 |
Diana-Olimpia Alexandrescu
Department of Mathematics, University of Craiova
200585 Craiova, Romania
email: alexandrescudiana@yahoo.com
|
Return to the EJDE web page
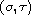 differentiable functions and studied the Riemann generalized derivatives
In this article we study the convexity and monotonicity of
differentiable functions and studied the Riemann generalized derivatives
In this article we study the convexity and monotonicity of
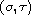 differentiable functions, using results by Hincin, Humke and Laczkovich,
and using the Riemann generalized derivative.
We give conditions such that the classic properties of differentiable
functions hold also for
differentiable functions, using results by Hincin, Humke and Laczkovich,
and using the Riemann generalized derivative.
We give conditions such that the classic properties of differentiable
functions hold also for
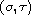 differentiable functions.
differentiable functions.


