We study the linear second-order differential equation
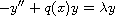
where, amongst other conditions,
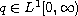 .
We obtain a convergent series expansion for the spectral
function which is valid for small values of
.
We obtain a convergent series expansion for the spectral
function which is valid for small values of
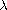 .
We also derive an asymptotic representation.
.
We also derive an asymptotic representation.
B. J. Harris
Abstract:
We study the linear second-order differential equation
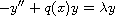
where, amongst other conditions,
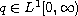 .
We obtain a convergent series expansion for the spectral
function which is valid for small values of
.
We obtain a convergent series expansion for the spectral
function which is valid for small values of
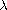 .
We also derive an asymptotic representation.
.
We also derive an asymptotic representation.
Submitted July 9, 2012. Published January 21, 2013.
Math Subject Classifications: 34E05.
Key Words: Sturm Liouville equation; spectral function;
small eigenparameter.
Show me the PDF file (172 KB), TEX file, and other files for this article.
 |
Bernie J. Harris Department of Mathematical Sciences Northern Illinois University DeKalb, IL 60115-2888, USA email: harris@math.niu.edu |
|---|
Return to the EJDE web page