Electron. J. Diff. Equ.,
Vol. 2013 (2013), No. 107, pp. 1-22.
Serrin blow-up criterion for strong solutions to the 3-D
compressible nematic liquid crystal flows with vacuum
Qiao Liu
Abstract:
In this article, we extend the well-known Serrin's blow-up criterion
for solutions of the 3-D incompressible Navier-Stokes equations to
the 3-D compressible nematic liquid crystal flows where the initial
vacuum is allowed. It is proved that for the initial-boundary value
problem of the 3-D compressible nematic liquid crystal flows in a
bounded domain, the strong solution exists globally if the velocity
satisfies the Serrin's condition and
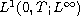 -norm of
the gradient of the velocity is bounded.
-norm of
the gradient of the velocity is bounded.
Submitted September 12, 2012. Published April 24, 2013.
Math Subject Classifications: 76A15, 76N10, 35B65, 35Q35
Key Words: Compressible nematic liquid crystal flows; strong solution;
Serrin's criterion; blow-up criterion;
compressible Navier-Stokes equations.
Show me the PDF file (301 KB),
TEX file, and other files for this article.
 |
Qiao Liu
College of Mathematics and Computer Science
Hunan Normal University, Changsha
Hunan 410081, China
email: liuqao2005@163.com
|
|---|
Return to the EJDE web page
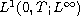 -norm of
the gradient of the velocity is bounded.
-norm of
the gradient of the velocity is bounded.
