Electron. J. Diff. Equ.,
Vol. 2012 (2012), No. 10, pp. 1-14.
Real interpolation spaces between the domain of the
Laplace operator with transmission conditions and L^p
on a polygonal domain
Aissa Aibeche, Wided Chikouche, Yasmina Daikh
Abstract:
We provide a description of the real interpolation spaces
between the domain of the Laplace operator (with transmission
conditions in a polygonal domain
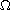 ) and
) and
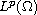 as
interpolation spaces between
as
interpolation spaces between
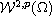 (possibly augmented with singular solutions) and
(possibly augmented with singular solutions) and
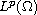 .
This result relies essentially on estimates on the resolvent
and the reiteration theorem.
.
This result relies essentially on estimates on the resolvent
and the reiteration theorem.
Submitted April 10, 2011. Published January 13, 2012.
Math Subject Classifications: 35R05, 35A20, 46B70.
Key Words: Transmission problem; non-smooth domains; interpolation.
Show me the PDF file (290 KB),
TEX file, and other files for this article.
 |
Aissa Aibeche
Université de Setif
Département de Mathéematiques
Faculté des Sciences
Setif 19000, Algeria
email: aibeche@univ-setif.dz
|
|---|
 |
Wided Chikouche
Université de Jijel
Laboratoire de Mathématiques Pures et Appliquées
B.P. 98, Ouled Aissa,
18000 Jijel, Algeria
email: w_chikouche@yahoo.com
|
|---|
 |
Yasmina Daikh
Université de Jijel
Laboratoire de Mathématiques Pures et Appliquées
B.P. 98, Ouled Aissa,
18000 Jijel, Algeria
email: yasmina_daikh@yahoo.fr
|
|---|
Return to the EJDE web page
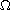 ) and
) and
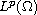 as
interpolation spaces between
as
interpolation spaces between
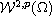 (possibly augmented with singular solutions) and
(possibly augmented with singular solutions) and
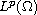 .
This result relies essentially on estimates on the resolvent
and the reiteration theorem.
.
This result relies essentially on estimates on the resolvent
and the reiteration theorem.


