The symmetry breaking phenomenon (SBP) to the Henon equation was first numerically observed in [4] and then theoretically verified on the unit ball
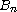 in [8]. Some
results on the asymptotic behavior of the ground states to the
Henon equation on
in [8]. Some
results on the asymptotic behavior of the ground states to the
Henon equation on
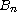 are presented in [2,3,7].
[8] further discussed SBP to
the p-Henon equation and obtained some results with
special value
are presented in [2,3,7].
[8] further discussed SBP to
the p-Henon equation and obtained some results with
special value
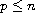 on
on
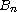 .
To inspire theoretical study on
more general p, a series of numerical experiments to the
p-Henon equation on a disk and a square are carried out
in this paper. Numerical computations are made by the minimax method
developed in [9,10]. Then, SBP, a peak break
phenomenon (PBP); i.e., a 1-peak solution, which is symmetric about
two axes and two diagonal lines, breaks its peak from 1 to 4, and a
1-peak positive non-ground state solution, which is only symmetric
about one axis, on the square are numerically captured and
visualized. The peak point and the peak height of the ground states
are carefully calculated to study their asymptotic behavior. Several
conjectures are made based on the numerical observations to
stimulate theoretical analysis. Two of them are proved in this paper.
.
To inspire theoretical study on
more general p, a series of numerical experiments to the
p-Henon equation on a disk and a square are carried out
in this paper. Numerical computations are made by the minimax method
developed in [9,10]. Then, SBP, a peak break
phenomenon (PBP); i.e., a 1-peak solution, which is symmetric about
two axes and two diagonal lines, breaks its peak from 1 to 4, and a
1-peak positive non-ground state solution, which is only symmetric
about one axis, on the square are numerically captured and
visualized. The peak point and the peak height of the ground states
are carefully calculated to study their asymptotic behavior. Several
conjectures are made based on the numerical observations to
stimulate theoretical analysis. Two of them are proved in this paper.