Electron. J. Diff. Equ.,
Vol. 2011 (2011), No. 134, pp. 1-15.
Longitudinal librations of a satellite
Massimo Furi, Mario Martelli, Alfonso Vignoli
Abstract:
Furi, Martelli and Landsberg gave a theoretical
explanation of the chaotic longitudinal librations of Hyperion,
a satellite of Saturn. The analysis was made under the simplifying
assumption that the spin axis remains perpendicular to the orbit
plane. Here, under the same assumption, we investigate the behavior
of the longitudinal librations of any satellite. Also we show that
they are possibly chaotic depending on two parameters: a constant
k related to the principal moments of inertia of the satellite,
and the eccentricity e of its orbit.
We prove that the plane k-e contains an open region
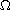 with the property that the longitudinal librations of any satellite
are possibly chaotic if the point (k,e) belongs to this region.
Since Hyperion's point is inside
with the property that the longitudinal librations of any satellite
are possibly chaotic if the point (k,e) belongs to this region.
Since Hyperion's point is inside
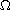 ,
the results of this paper
are more general than those obtained previously.
,
the results of this paper
are more general than those obtained previously.
Submitted April 18, 2011. Published October 14, 2011.
Math Subject Classifications: 34C28, 70F15.
Key Words: Ordinary differential equations;
Chaotic behavior of a satellite; librations.
Show me the PDF file (343 KB),
TEX file, and other files for this article.
 |
Massimo Furi
Dipartimento di Matematica Applicata
Università degli Studi di Firenze
Via S. Marta 3, 50139 Firenze, Italy
email: massimo.furi@unifi.it
|
|---|
 |
Mario Martelli
Department of Mathematics
Claremont Graduate University
710 N College Ave
Claremont, CA 91711, USA
email: mario.martelli@cgu.edu
|
|---|
 |
Alfonso Vignoli
Dipartimento di Matematica
Università di Roma Tor Vergata
Via della Ricerca Scientifica 1,
00133, Roma, Italy
email: vignoli@mat.uniroma2.it
|
|---|
Return to the EJDE web page
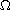 with the property that the longitudinal librations of any satellite
are possibly chaotic if the point (k,e) belongs to this region.
Since Hyperion's point is inside
with the property that the longitudinal librations of any satellite
are possibly chaotic if the point (k,e) belongs to this region.
Since Hyperion's point is inside
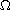 ,
the results of this paper
are more general than those obtained previously.
,
the results of this paper
are more general than those obtained previously.


