Electron. J. Diff. Equ.,
Vol. 2011 (2011), No. 119, pp. 1-10.
Estimates and uniqueness for boundary blow-up solutions of
p-Laplace equations
Monica Marras, Giovanni Porru
Abstract:
We investigate boundary blow-up solutions of the p-Laplace
equation
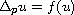 ,
,
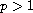 ,
in a bounded smooth
domain
,
in a bounded smooth
domain
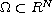 .
Under appropriate conditions on the
growth of
.
Under appropriate conditions on the
growth of
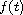 as
as
 approaches infinity, we find an estimate
of the solution
approaches infinity, we find an estimate
of the solution
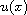 as
as
 approaches
approaches
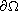 ,
and a uniqueness result.
,
and a uniqueness result.
Submitted November 21, 2010. Published September 15, 2011.
Math Subject Classifications: 35B40, 35B44, 35J92.
Key Words: p-Laplace equations; large equations; uniqueness;
second order boundary approximation.
Show me the PDF file (220 KB),
TEX file, and other files for this article.
 |
Monica Marras
Dipartimento di Matematica e Informatica
Universitá di Cagliari
Via Ospedale 72, 09124 Cagliari, Italy
email: mmarras@unica.it
|
|---|
 |
Giovanni Porru
Dipartimento di Matematica e Informatica
Universitá di Cagliari
Via Ospedale 72, 09124 Cagliari, Italy
email: porru@unica.it
|
|---|
Return to the EJDE web page
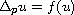 ,
,
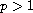 ,
in a bounded smooth
domain
,
in a bounded smooth
domain
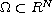 .
Under appropriate conditions on the
growth of
.
Under appropriate conditions on the
growth of
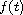 as
as
 approaches infinity, we find an estimate
of the solution
approaches infinity, we find an estimate
of the solution
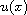 as
as
 approaches
approaches
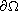 ,
and a uniqueness result.
,
and a uniqueness result.

